Competency Based Questions for Class 10 Maths Chapter 7 Coordinate Geometry

Competency Based Questions are new type of questions asked in CBSE Board exam for class 10. Practising the following Competency Based Questions will help the students in facing Board Questions.

Hint: Identify x and y coordinate in order to plot points on the graph.

Question.1. Sheena was asked to plot a point 10 unit on the left of the origin and other point 4 units directly above the origin. Which of the following are the two points?

(a) (10,0) and (0,-4)
(b) (-10,0) and (4,0)
(c) (10,0) and (0,4)
(d) (-10, 0) and (0, 4)

Answer. (d) (-10, 0) and (0, 4)

Question.2. Three points lie on a vertical line. Which of the following could be those points?

(a) (0, 4), (4, 0), (0, 0)
(b) (4, 3), (5, 3), (-12, 3)
(c) (-8, 7), (-8,-8), (-8, -100)
(d) (-8,3), (-8, 8), (8,7)

Answer. (c) (-8, 7), (-8,-8), (-8, -100)

Hint: Apply and derive distance formula in order to determine the distance between two coordinates on the graph.

Question.3. On a graph, two-line segments, AB and CD of equal length are drawn. Which of these could be the coordinates of the points, A, B, C and D?

(a) A(-3,4) B(-1,2) and C(3,4) D(1,2)
(b) A(-3,-4) B(-1,2) and C(3,4) D(1,2)
(c) A(-3,4) B(-1,-2) and C(3,4) D(1,2)
(d) A(3,4) B(-1,2) and C(3,4) D(1,2)

Answer. (a) A(-3,4) B(-1,2) and C(3,4) D(1,2)

Question.4. The distance between two points, M and N, on a graph is given as \sqrt{10^{2}+7^{2}}. The coordinates of point M are (–4. 3). Given that the point N lies in the first quadrant, which of the following is true about the all possible x-coordinates of point N?

(a) They are multiple of 2.
(b) They are multiples of 3.
(c) They are multiples of 5.
(d) They are multiples of 6.

Answer. (b) They are multiples of 3.

Hint: Apply distance formula in order to solve various mathematical and real-life situations graphically.

Question.5. On a coordinate grid, the location of a bank is (–4, 8) and the location of a post office is (2, 0). The scale used is 1 unit = 50 m. What is the shortest possible distance between the bank and the post office?

(a) 200 m
(b) 500 m
(c) 700 m
(d) 800 m

Answer. (b) 500 m

Question.6. The graph of a circle with centre O with point R on its circumference is shown. 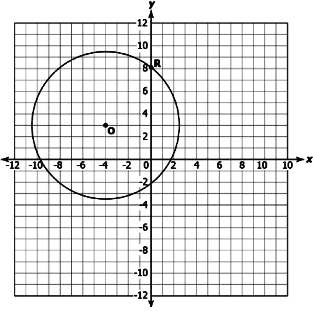What is the side length of the square that circumscribes the circle?

(a) 2 \sqrt{41} Units
(b) \sqrt{41} Units
(c) 3 \sqrt{17} Units
(d) 6 \sqrt{17} Units

Answer. (a) 2 \sqrt{41} Units

Hint: Apply and derive section formula in order to divide the line segment in a given ratio.

Question.7. A point G divides a line segment in the ratio 3:7. The segment starts at the origin and ends at a point K having 20 as its abscissa and 40 as its ordinate. Given that G is closer to the origin than to point K, which of the following are the coordinates of point G?

(a) (14, 28)
(b) (28, 14)
(c) (12, 6)
(d) (6, 12)

Answer. (d) (6, 12)

Question.8. Two poles are to be installed on an elevated road as shown in the diagram. 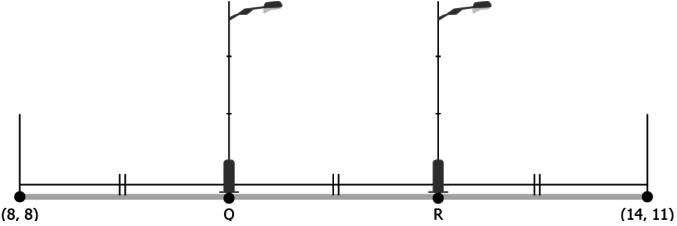The diagram also shows the starting and ending points of the road. Which of the following are the coordinates of the poles?

(a) Q (10,9) and R(12,8)
(b) Q(10,8) and R (12,11)
(c) Q (10,9) and R(12,10)
(d) Q(-10, 9) and R(0, 11)

Answer. (c) Q (10,9) and R(12,10)

Hint: Apply distance and section formula in order to determine the vertices/ diagonals/ mid points of given geometrical shapes.

Question.9. Which of the following are the coordinates of the intersection points of the diagonals of the rectangle ABCD with vertices A(0,3), B(3,0), C(1,-2) and D(-2,1)?

(a) (1.5, 1.5)
(b) \left(\frac{1}{2},\frac{1}{2} \right)
(c) \left(-\frac{1}{2},-\frac{1}{2} \right)
(d) (2, -1)

Answer. (b) \left(\frac{1}{2},\frac{1}{2} \right)

Question.10. The figure shows a parallelogram with one of its vertices intersecting the y-axis at 3 and another vertex intersecting the x-axis at 2. 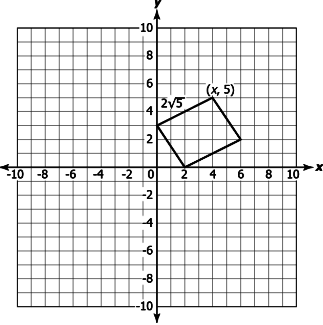If (m, n) is the intersection point of the diagonals of the parallelogram, which relation is correct?

(a) m = 0.5 + n
(b) m = n – 0.5
(c) m = 1.50 + n
(d) m = n – 1.50

Answer. (a) m = 0.5 + n

Hint: Apply and derive the formula of area of triangle geometrically in order to determine the area of quadrilateral/triangle.

Question.11. A triangle is drawn on a graph. Two of the vertices of the triangle intersect the y-axis at -3 and x-axis at 5. The third vertex is at (2, 4). What is the area of the triangle?

(a) 16 square units
(b) 14.5 square units
(c) 8 square units
(d) 6.5 square units

Answer. (b) 14.5 square units

Question.12. Observe the triangles PMN and PQR shown below. The area of the triangle PQR is 14 square units.What is the area of the triangle PMN?

(a) 3.5 square units
(b) 7 square units
(c) 2.5 square units
(d) 1 square unit

Answer. (a) 3.5 square units