NCERT Solutions Class 10 Maths Chapter 1 Real Numbers
NCERT Solutions
Exercise 1.1
Question.1. Express each number as a product of its prime factors :
(i) 140
Solution: Factorising by Prime Factorization method. We get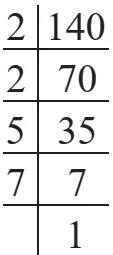
= 2^{2} \times 5 \times 7
(ii) 156
Solution: 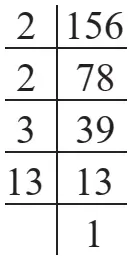
= 2^{2} \times 3 \times 13
(iii) 3825
Solution: 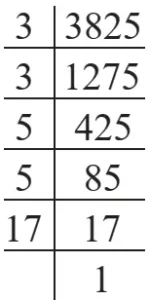
= 3^{2} \times 5^{2} \times 17
(iv) 5005
Solution: 
(v) 7429
Solution: 
Question.2. Find the L.C.M. and H.C.F. of the following pairs of integers and verify that L.C.M. \times H.C.F. = product of the two numbers
(i) 26 and 91
Solution: Prime factors of 26 = 2 \times 13
Prime factors of 91 = 7 \times 13
∵ H.C.F. = the product of the smallest power of common factors of the numbers
Hence, H.C.F. = 13
∵ L.C.M. = the product of the greatest power of each prime factors associated with the numbers.
Hence, L.C.M. = 2 \times 7 \times 13 = 182
Now, H.C.F. \times L.C.M. = 13 \times 182 = 2366
and product of both the numbers = 26 \times 91 = 2366
Hence, L.C.M. \times H.C.F. = product of the two numbers
(ii) 510 and 92
Solution: Prime factors of 510 = 2 \times 3 \times 5 \times 17
Prime factor of 92 = 2 \times 2 \times 23 = 2^{2} \times 23
∵ H.C.F. = the product of the smallest power of common factors of the numbers
Hence, H.C.F. = 2
∵ L.C.M. = the product of the greatest power of each of the prime factors associated with the numbers.
Hence, L.C.M. = 2^{2} \times 3 \times 5 \times 17\times 23 = 23460
Now, H.C.F. \times L.C.M. = 2 \times 23460 = 46920
and Product of two numbers = 510 \times 92 = 46920
Hence, L.C.M. \times H.C.F. = product of the two numbers
(iii) 336 and 54
Solution: Prime factors of 366 = 2^{4} \times 3 \times 7
Prime factors of 54 = 2 \times 3^{3}
∵ H.C.F. = the product of the smallest power of common factors of the numbers
= 2 \times 3 = 6
∵ L.C.M. = The product of the greatest power of each of the prime factors associated with the numbers.
= 2^{4} \times 3^{3} \times 7 = 3024
Now, H.C.F. \times L.C.M.= 6 \times 3024 = 18144
and Product of two numbers 336 \times 54 = 18144
Hence, L.C.M. \times H.C.F. = product of the two numbers
Question.3. Find the LCM and HCF of the following integers by applying the prime factorisation method.
(i) 12, 15 and 21
Solution: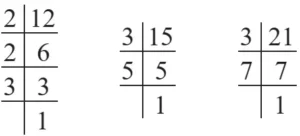
15 = 3 \times 5
21 = 3 \times 7
∴ H.C.F. = 3
and L.C.M. = 2^{2} \times 3 \times 5 \times 7 = 420
(ii) 17, 23 and 29
Solution: 17, 23 and 29 are prime numbers.
∴ H.C.F. = 1
and L.C.M. = 17 \times 23 \times 29 = 11,339
(iii) 8, 9 and 25
Solution: 
9 = 3^{2} ,
25 = 5^{2}
∴ H.C.F. = 1
and L.C.M. = 2^{3} \times 3^{2} \times 5^{2} = 1800
Question.4. Given that H.C.F. (306, 657) = 9, find L.C.M. (306, 657).
Solution: Given, H.C.F. (306, 657) = 9
Given numbers = 306, 657
∴ H.C.F. (306, 657) \times L.C.M. (306, 657) = Product of two numbers
9 \times L.C.M. (306, 657) = 306 \times 657
L.C.M. (306, 657) = \frac{306 \times 657}{9}
= 306 \times 73
L.C.M. (306, 657) = 22,338
Question.5. Check whether 6^{n} can end with the digit 0 for any natural number n.
Solution: If unit digit of any number is 0 then it is always divisible by 5.
Let unit digit of 6^{n} is 0 then it should be divided by 5, it is only possible when one of the prime factors of 6^{n} is 5.
Now, 6 = 2 × 3
6^{n} = (2 \times 3)^{n}
= 2^{n} \times 3^{n}
Thus, 5 is not present in the factors of 6^{n} . Hence, there is no value for n ∈ N for which unit digits is 0.
Question.6. Explain why 7 \times 11 \times 13 + 13 and 7 \times 6 \times 5 \times 4 \times 3 \times 2 \times 1 + 5 are composite numbers.
Solution: (i) Given, 7 \times 11 \times 13 + 13
= 13 \times (7 \times 11 + 1)
= 13 \times (77 + 1)
= 13 \times 78
= 13 \times 13 \times 3 \times 2
It means factors of given expression are 2, 3 and 13 respectively. Hence, it is composite number.
(ii) 7 \times 6 \times 5 \times 4 \times 3 \times 2 \times 1 + 5
= 5(7 \times 6 \times 4 \times 3 \times 2 + 1)
= 5 \times (1008 + 1)
= 5 \times 1009
Factors of given expression is 5 and 1009. Hence, it is composite number.
Question.7. There is a circular path around a sports field. Sonia takes 18 minutes to drive one round of the field, while Ravi takes 12 minutes for the same. Suppose they both start at the same point and at the same time, and go in the same direction. After how many minutes will they meet again at the starting point?
Solution: Sonia takes time to drive one round = 18 min
Ravi takes time to drive one round = 12 min
If they both start at the same point and at the same time and go in the same direction. To find out the again meeting time at the starting point we need to find out the L.C.M. of 18 and 12.
So, 18 = 2 \times 3^{2}
12 = 2^{2} \times 3
L.C.M. = Product of the greatest power of each prime factors
= 22 \times 32 = 9 \times × 4 = 36
Thus, they will meet after 36 min.
Exercise 1.2
Question.1. Prove that \sqrt{5} is an irrational number.
Solution: Let \sqrt{5} is a rational number. It can be written as form \frac{a}{b} , where a and b are co-prime number.
Hence, \sqrt{5} = \frac{a}{b}
⇒ (\sqrt{5})^{2} = (\frac{a}{b})^{2}
⇒ 5 = \frac{a^{2}}{b^{2}}
⇒ 5b^{2} = a^{2} ………(i)
a^{2} is divided by 5 then a is divided by 5.
Then we can write a = 5m, where m is any integer
Put a = 5m in equation (i),
5b^{2} = (5m)^{2}
⇒ 5b^{2} = 25m^{2}
⇒ b^{2} = 5m^{2}
b^{2} is divided by 5, then b is also divided by 5. So, a and b have atleast one common factor but this contradicts the fact that a and b are co-prime numbers. We got this contradiction because we have mistakenly assumed that 5 is a rationl number.
Hence, it concludes that \sqrt{5} is an irrational number.
Question.2. Prove that 3 +2 \sqrt{5} is an irrational.
Solution: Let 3 +2 \sqrt{5} is a rational number. It can be written as form \frac{a}{b} ,
where a and b are co-prime numbers.
So, 3 + 2 \sqrt{5} = \frac{a}{b}
⇒ 2 \sqrt{5} = \frac{a-3b}{b}
⇒ \sqrt{5} = \frac{a-3b}{2b}
∵ a and b are integers.
∴ \frac{a-3b}{2b} is a rational number.
It means \sqrt{5} is also rational number but we know that \sqrt{5} is an irrational number. Hence, our assumption 3 +2 \sqrt{5} is a rational number is wrong.
Hence, 3 +2 \sqrt{5} is an irrational number.
Question.3. Prove that the following numbers are irrational :
(i) 7 \sqrt{5}
Solution: Let 7 \sqrt{5} is a rational number which is in the form of \frac{a}{b} .
Where, b ≠ 0
7 \sqrt{5} = \frac{a}{b}
Squaring on both sides,
⇒ 245 = \frac{a^{2}}{b^{2}}
⇒ a^{2} = 245b^{2} …..(i)
a^{2} is divisible by 245
then a will be divisible by 245
Let, a = 245m
By equation (i),
⇒ (245m)^{2} = 245b^{2} ……(ii)
∴ b^{2} is divisible by 245
then b will be divided by 245
by equation (i) and (ii), the common factor of a and b is 245. Which contradicts our assumption that a and b do not have any common factor other than 1. Hence, 7 \sqrt{5} is irrational number.
(ii) 6 + \sqrt{2}
Solution: Let 6 + \sqrt{2} is a rational number.
So, it can be written as form a \frac{a}{b}
6 + \sqrt{2} = \frac{a}{b} where a, b are co-prime numbers and b ≠ 0.
⇒ \sqrt{2} = \frac{a}{b} – 6
⇒ \sqrt{2} = \frac{a-6b}{b}
∴ a, b and 6 are integers. So \frac{a-6b}{b} is a rational number. But by the contradiction method. \sqrt{2} is an irrational number. Hence, 6 + \sqrt{2} is an irrational number.
(iii) \frac{1}{ \sqrt{2}}
Solution: Let \frac{1}{ \sqrt{2}} is a rational it can be written as form \frac{a}{b} .
\frac{1}{ \sqrt{2}} = \frac{a}{b}
\frac{b}{a} = \sqrt{2}
\frac{b}{a} is a rational number but this contradicts the fact that \sqrt{2} is an irrational number.
Thus, \frac{1}{ \sqrt{2}} is an irrational number.




